This video shows how to calculate and classify the critical points of functions of two variables.

How to find critical points of a function. A critical point of a multivariable function is a point where the partial derivatives of first order of this function are equal to zero. Steps for finding the critical points of a given function f (x): What are the critical numbers of a function?

Find the critical points of {eq}f (x) {/eq} by equating the first derivative to zero. 1.) take derivative of f (x) to get f ‘ (x) 2.) find x values where f ‘ (x) = 0 and/or where f ‘ (x) is undefined. The main point of this section is to work some.

Well, the critical numbers of a function are values of the function {eq}f (x) {/eq}, x=c, such that either. 3.) plug the values obtained. Next, find all values of the function's independent.

To find the critical points of a function, first ensure that the function is differentiable, and then take the derivative. Given a function f(x), a critical point of the function is a value x such that f'(x)=0. Examples with detailed solution on how to find the critical.

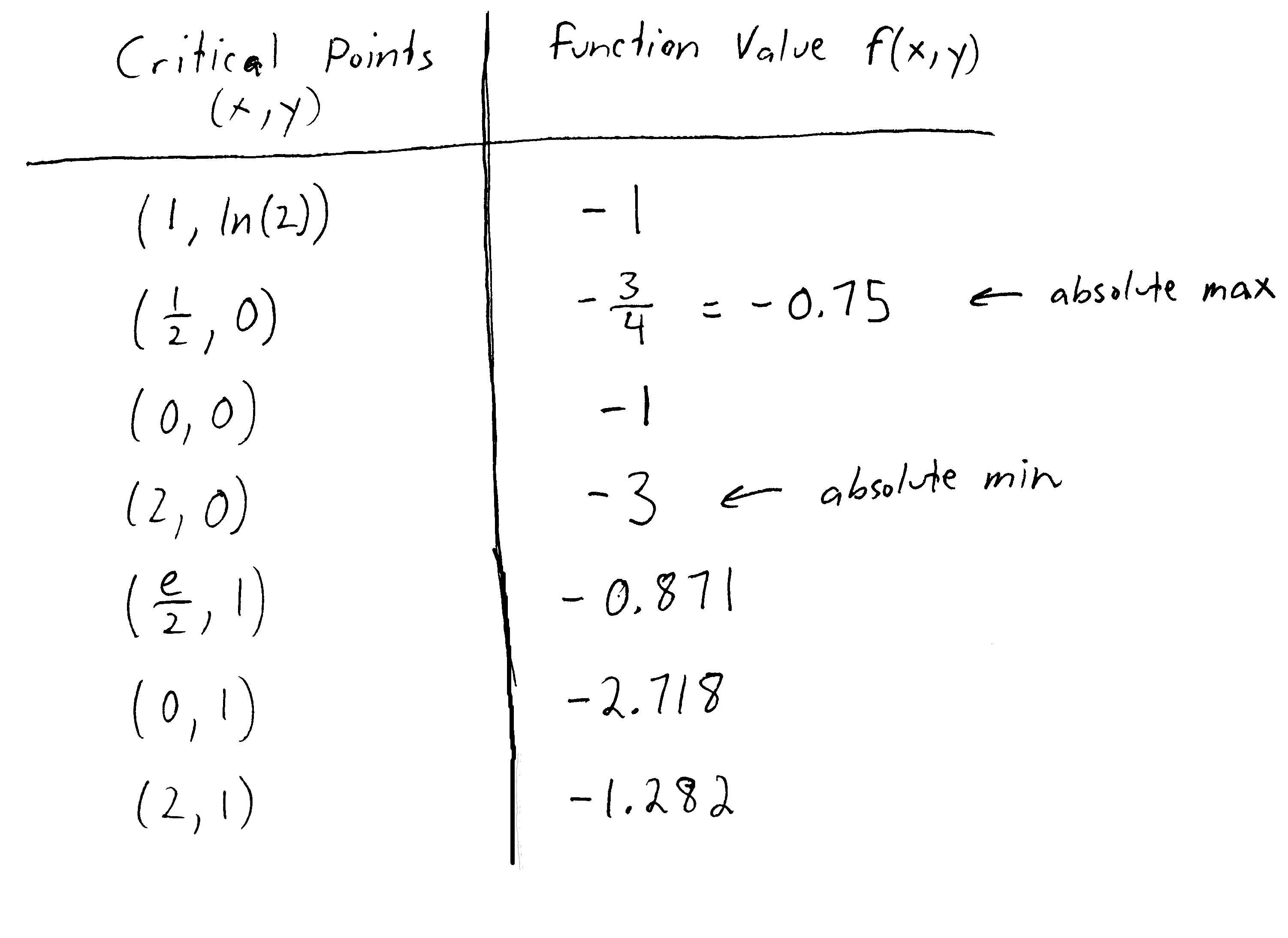
Find critical points of multivariable functions. Calculus with complex numbers is beyond the scope of this course and is usually taught in higher level mathematics courses. Since ϕ assumes as well positive as negative values in the immediate neighborhood of 0 we can.

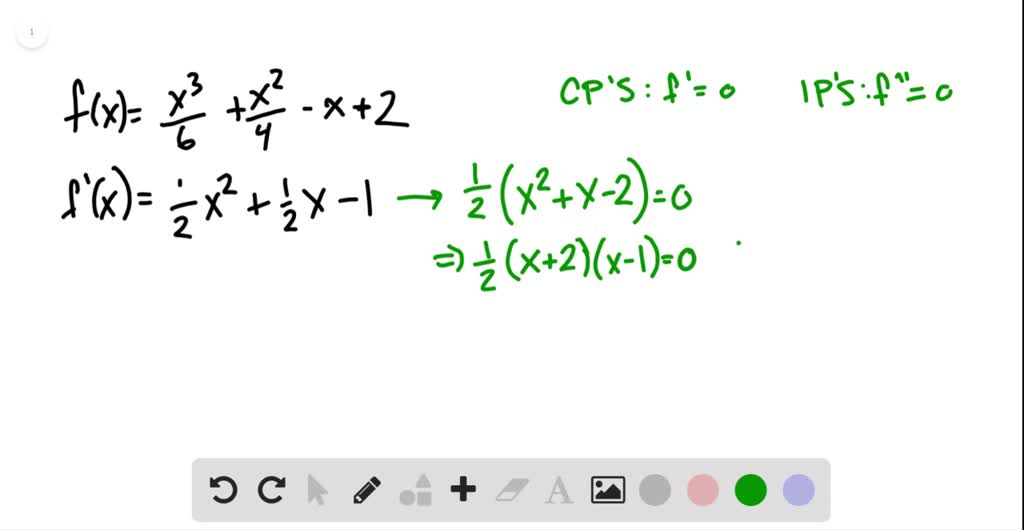
The critical point of the function of a single variable: This function has critical points at x = 1 x = 1 x = 1 and x = 3 x = 3 x = 3. The ideas involve first and second order derivatives and are seen in university mathematics.