The optimization process is all about finding a function’s least and greatest values.

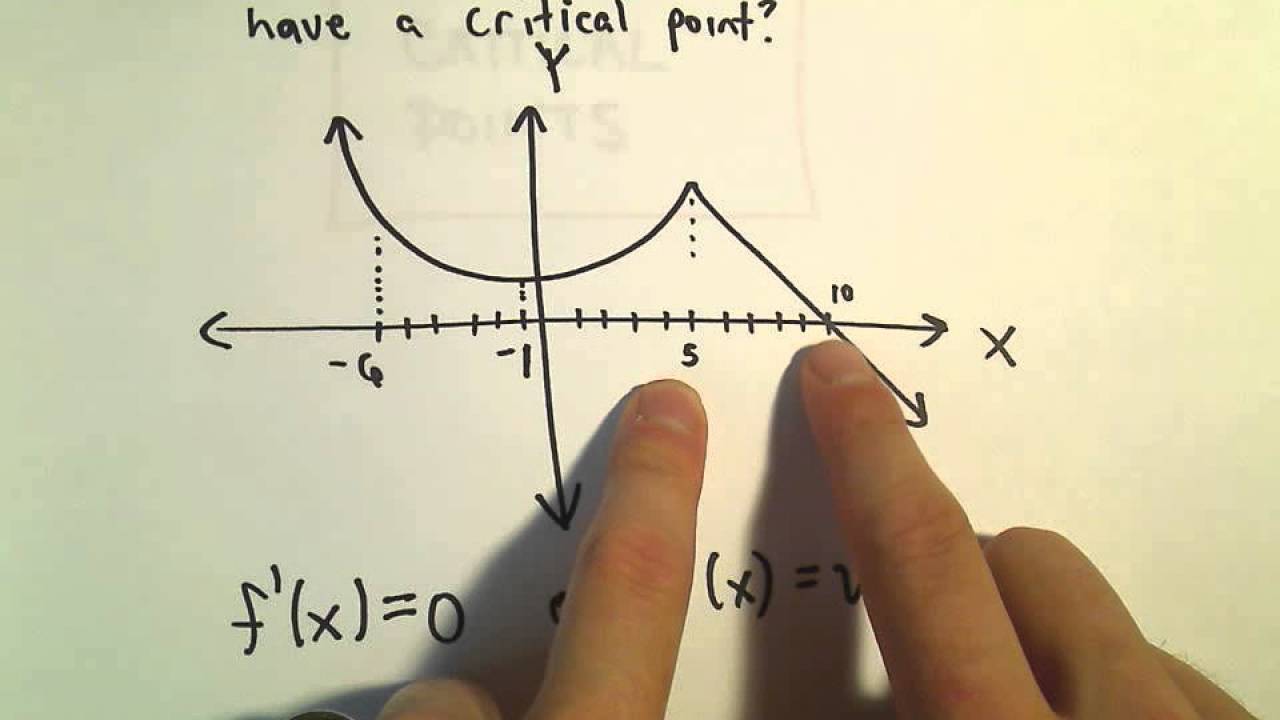
Critical points on graph. A critical point of a function of a single real variable, f ( x ), is a value x0 in the domain of f where it is not differentiable or its derivative is 0 ( f ′ ( x0) = 0). Critical points are points on a graph in which the slope changes sign (i. Just a quick example of fi.

Calculus with complex numbers is beyond the scope of this course and is usually taught in higher level mathematics courses. A local minimum if the function changes from decreasing to. The critical points of this graph are obvious, but if there were a complex graph, it would be convenient if i can get the graph to pinpoint the critical points.

Find critical point by graph observation. There have lots of peaks and. Critical points are points on a graph in which the slope changes sign (i.e.

Critical points are the points on the graph where the function’s change in rate is altered. Thanks to all of you who support me on patreon. These points exist at the very top or bottom of ‘humps’ on a graph.

As the complexity of the functions increase, we see more and more complex behavior from their graphs, and it becomes harder to graph. If we use a calculator to sketch the graph of a function, we can usually spot the least and. At , the first derivative is , and so is the slope of the tangent line.at , the derivative and the slope are both.at , the line is horizontal, demonstrating that the derivative at this point.

In most cases, though, the graph method will not be convenient because most functions are in expressions than graphs. 👉 learn the basics to graphing sine and cosine functions. This function has critical points at x = 1 x = 1 x = 1 and x = 3 x = 3 x = 3.