What is the maximum number of vertical.

What is vertical asymptote of a function. An asymptote is a line being approached by a curve but never touching the curve. How to find the vertical asymptotes of a function? Observe any restrictions on the domain of the function.

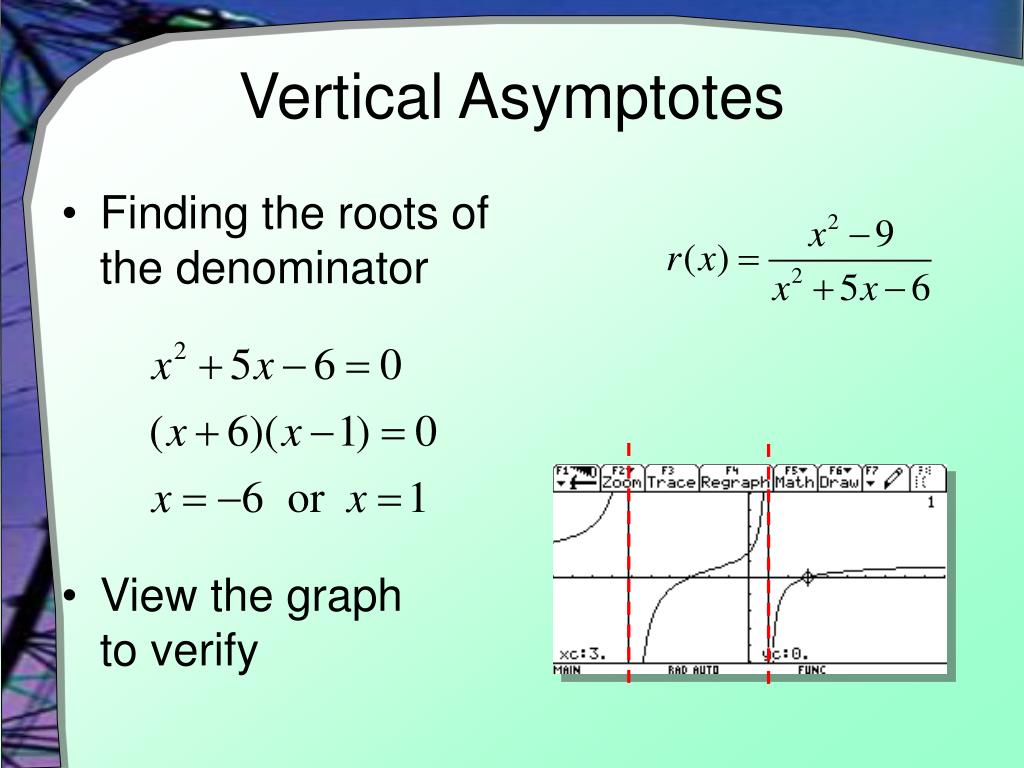
In the above example, we. According to my text, a reliable method of finding the asymptote is to factor the numerator and. Write out the function and make the denominator of the function having x = 0 solve for x in the denominator.

Here are the two steps to follow. 2) an example in which factors cancel and that has. A graphed line will bend and curve to avoid this region of the graph.

It explains how to distinguish a vertical asymptote from a hole and h. A graph can approach a horizontal asymptote in many different ways see figure 8 in §1.6 of the text for graphical illustrations. For functions with polynomial numerator and denominator, horizontal.

The graph may cross it but eventually, for large enough or. An asymptote is a horizontal/vertical oblique line whose distance from the graph of a function keeps decreasing and approaches zero but never gets there. We define an asymptote as a straight line that can be horizontal, vertical or obliquous that goes closer and closer to a curve which is the graphic of a given.

1) an example with two vertical asymptotes. I.e., an asymptote is a line to which the graph of a function converges. Finding the vertical asymptote function simply is explained in the following steps.