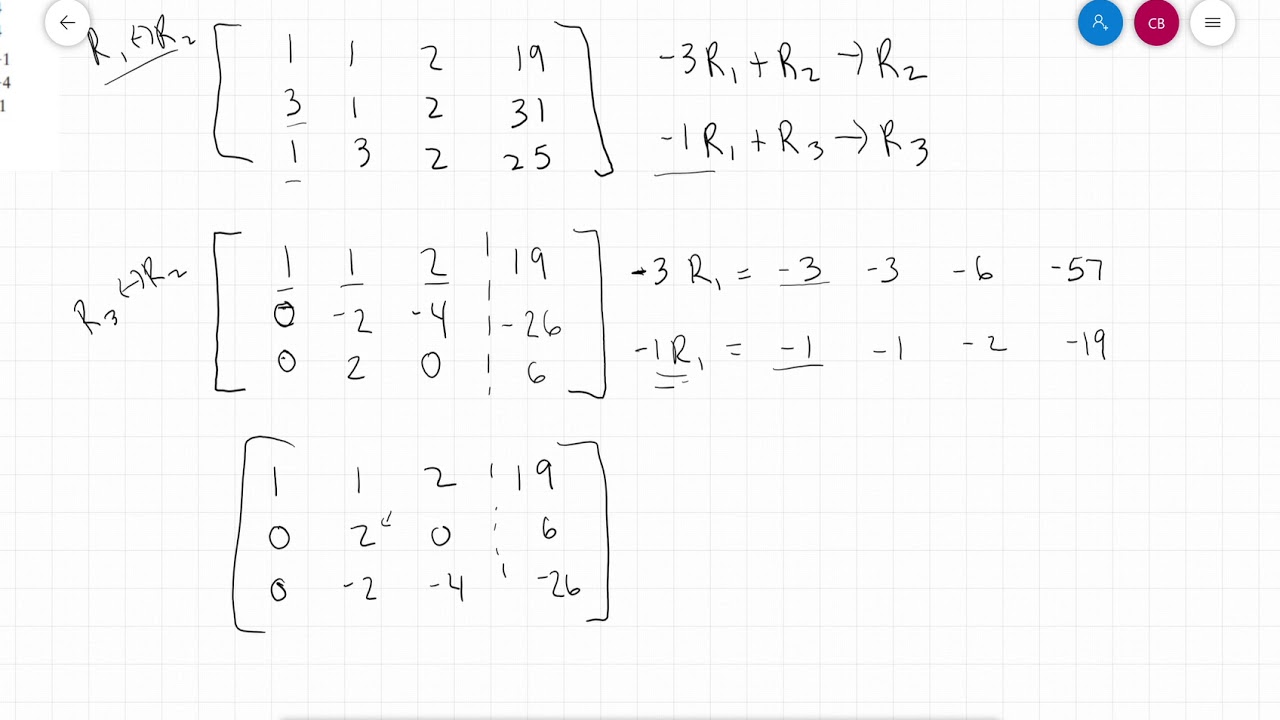
The idea of the row echelon form is to construct systematically an equivalent matrix via the use of invertible elementary matrices so get to a row echelon form,.

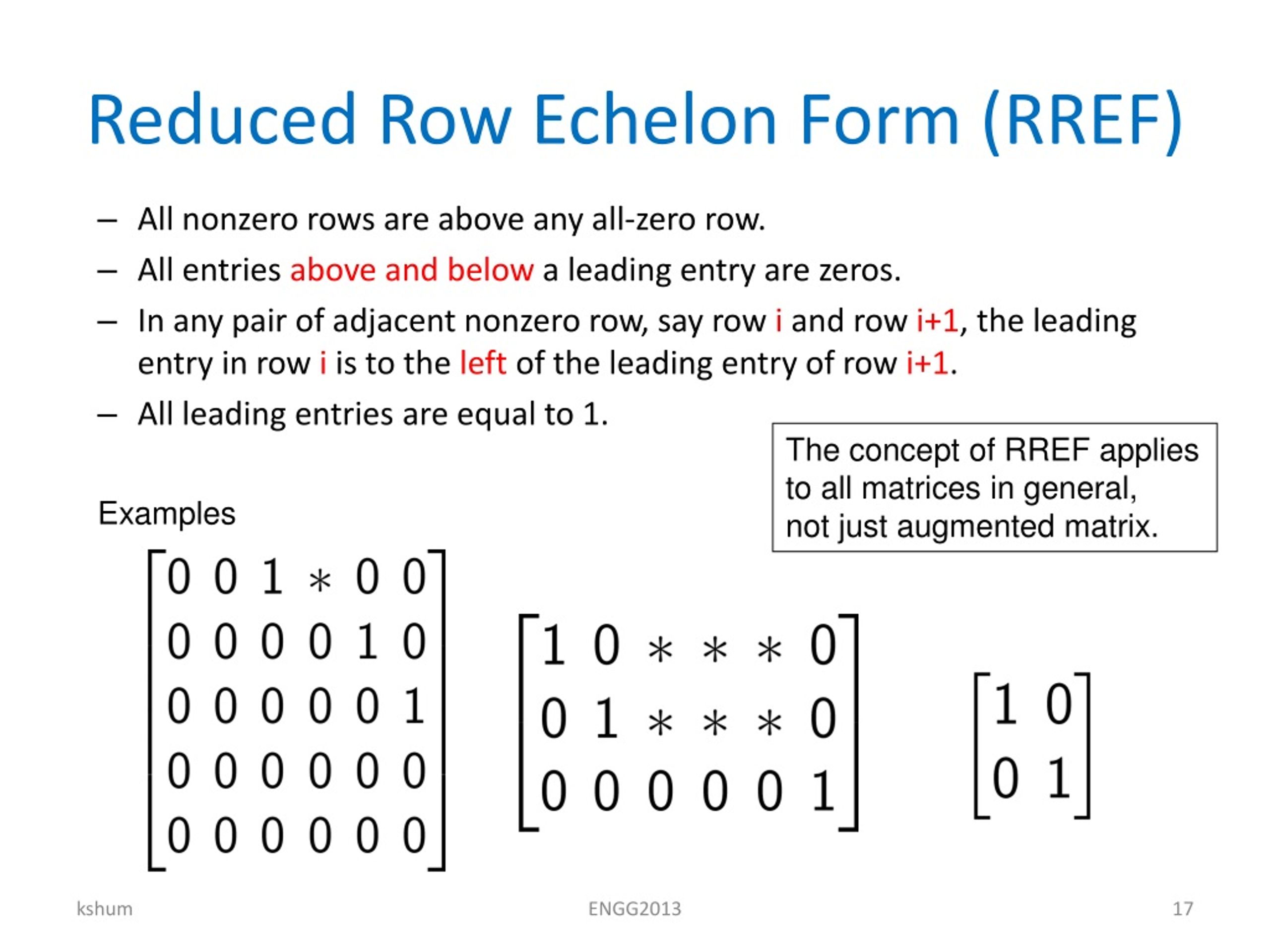
What is rref. Reduced row echelon form is also called row canonical. Each nonzero row after the first has a leading entry that is to the right of the previous row’s leading entry. As soon as it is changed into the reduced row.

In some of the situations when our text fails to define rref, so rref function makes it able to define. The reduced row echelon form of a matrix a is another matrix h that satisfies the following properties: It is the abbreviation of reduced row echelon form.

Understanding the difference between rref and ref determining whether each of the following matrices are in ref, rref, or neither. All zero rows are at the bottom of the matrix, hence the zero matrix is rref. A matrix is in reduced row echelon form (also called row canonical form) if it satisfies the following conditions:

What is reduced row echelon form (rref) of a matrix? Converting a matrix to rref (reduced row echelon form) makes solutions to linear systems of equations simpler to find. A) a) circle all that apply:

Row reduced echelon form (matrix algebra terminology) rref: It makes the lives of people who use matrices easier. [3] it is in row echelon form.

Rref(a) it returns reduced row echelon form r and a vector of pivots p; R = rref (a,tol) specifies a pivot tolerance that the algorithm uses to determine. There is another form that a matrix can be in, known as reduced.