We have to prove that:

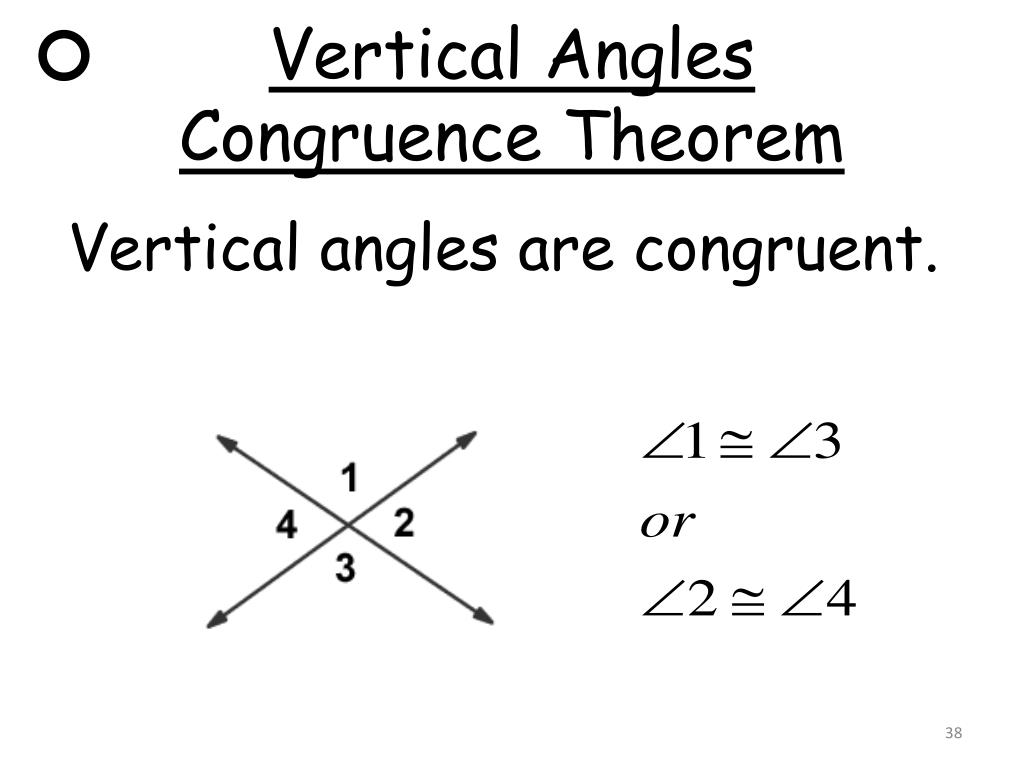
Vertical angles are congruent. Consider the two lines ab and cd intersecting each other at the point o. Also, a vertical angle and its adjacent angle are supplementary angles, i.e., they add up to 180 degrees. What i want to do in this video is prove to.

The vertical angles are of equal measurements. Vertical angle congruence theorem example. Vertical angles are angles in opposite corners of intersecting lines.

Both pairs of vertical angles (4 angles that share the same vertex) always add up to 360°. And thus you can set their measures equal to each other: Vertical angles share the same vertex (the common corner point) but they cannot share a side.

Vertical angles are congruent proof. The vertical angles are of equal measurements. Whenever an angle is bisected, two congruent angles are formed.

Name the angle vertical to \angle\textbf {5} ∠5. This theorem says that when two straight lines intersect,. According to the vertical angles theorem, vertical angles are always congruent.

These angles are equal, and here’s the official theorem that tells you. Now you have a system of two equations and two unknowns. This is the currently selected item.