So we start with differentiating :

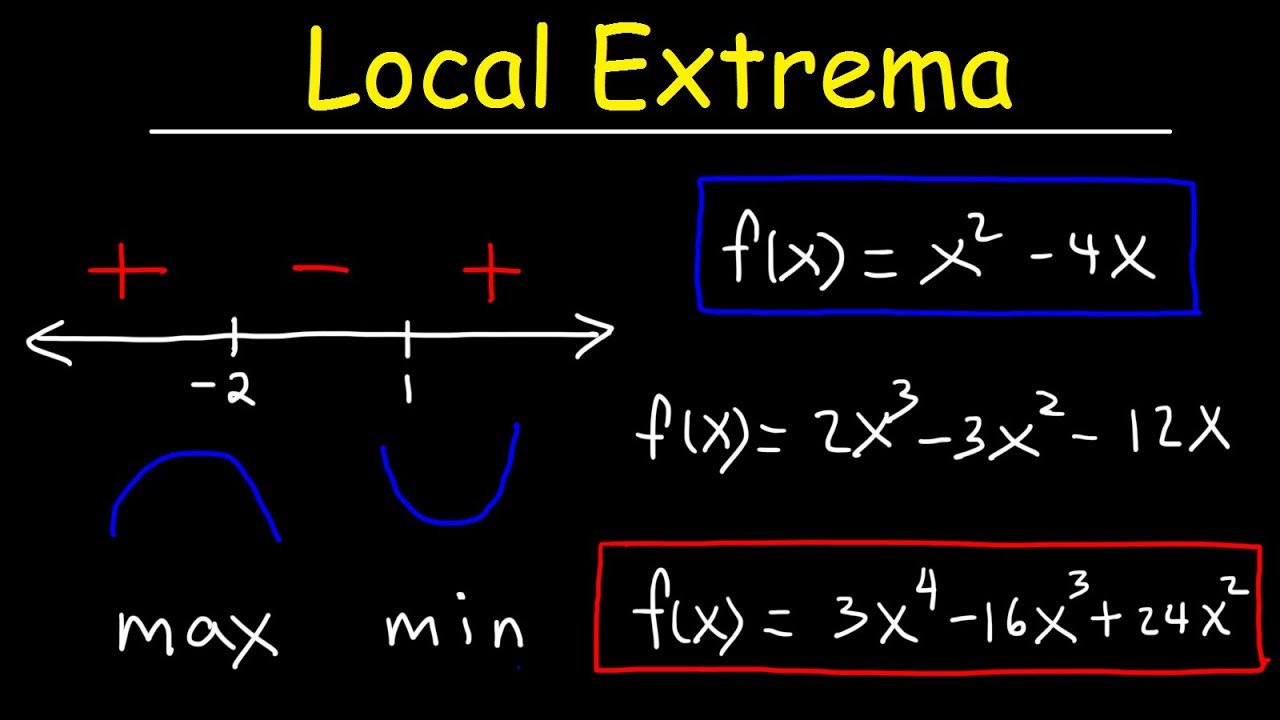
How to find relative extrema of a function. Example 1 determine the absolute extrema for the following function and interval. G(t) = 2t3 +3t2 −12t+4 on [−4,2] g ( t) = 2 t 3 + 3 t 2 − 12 t + 4. F ( x) = cos ( x) − e x.

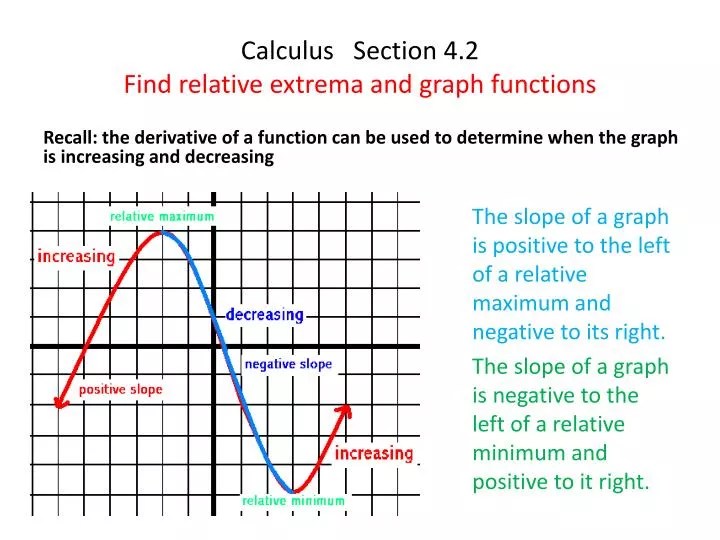
Find the locations of the. We will make use of: Peaks and valleys in a graph indicate the relative extrema of the function.

Given the function {eq}f(x) {/eq}, compute {eq}f'(x) {/eq}. This is the absolute maximum or minimum value over. If you want an accurate solution, for sure you need to use newton method.

To find the relative extremum points of , we must use. Observe that f ( x) does not have any relative extrema despite the fact that f ′ ( 0) = 0. The function has a relative maximum at (,) when.

Notice that f ′ ( x) does not change. The fact tells us that all relative extrema must be critical points so we know that if the function does have relative extrema then they must be in the collection of all the critical. This immediately tells us that to find the absolute extrema of a function on an interval, we need only examine the relative extrema inside the interval, and the endpoints of the interval.

Tour start here for a quick overview of the site help center detailed answers to any questions you might have meta discuss the workings and policies of this site Next, we will learn to identify the relative extrema of a function using a graph. Finding all critical points and all points where is.