Critical points are key in calculus to find maximum and minimum values of graphs.

How to find critical points on a graph. Let's say you bought a new dog, and went down to the local. The critical points of this graph are obvious, but if there were a complex graph, it would be convenient if i can get the graph to pinpoint the critical points. In most cases, though, the graph method will not be convenient because most functions are in expressions than graphs.

A local minimum if the function. Just a quick example of fi. A critical point can be a local maximum if the functions changes from increasing to decreasing at that point or.

Thanks to all of you who support me on patreon. For instance, they could let you know the lowest or lowest point of a suspension bridge (assuming you can plot the bridge. We say that x = c x = c is a critical point of the function f (x) f ( x) if f (c) f ( c) exists and if either of the following are true.

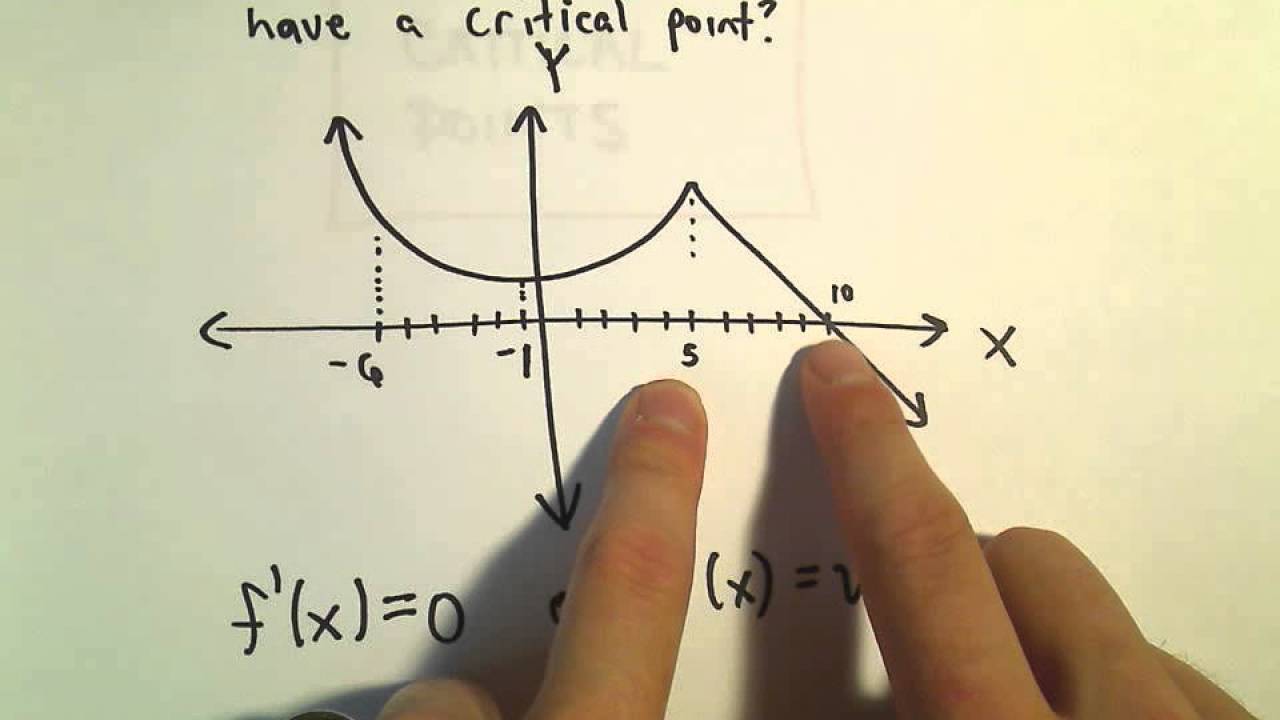
Note that we require that f (c) f ( c) exists in. Critical points are points on a graph in which the slope changes sign (i. How to find critical points on a graph find out the critical points for.

Critical points exist where the derivative is 0, and represent points at which the graph of the function changes direction from decreasing to increasing, vice versa. Crucial points in calculus have other applications, too.