The calculator can find derivatives using the sum rule, the elementary power rule, the generalized power rule, the reciprocal rule (inverse function rule), the product rule, the chain rule and.

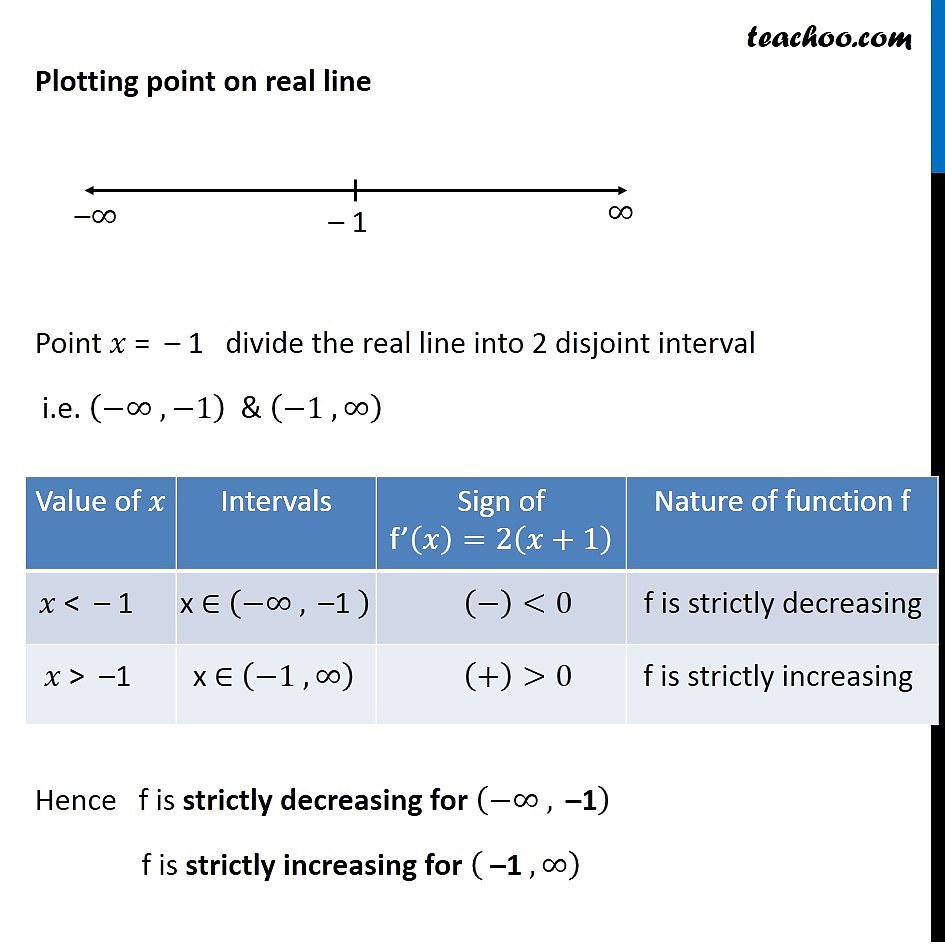
Function increasing or decreasing calculator. Increasing and decreasing functions calculator find the intervals on which f is increasing or decreasing calculator find where increasing and decreasing calculator A function is decreasing if the {eq}y {/eq} values continuously decrease as the {eq}x {/eq} values increase. Given a function, f (x), we can determine the intervals where it is increasing and decreasing by using differentiation and algebra.

F (x) = √x f ( x) = x. Now, taking out 3 common from the. For this particular function, use the power rule:

Graph the polynomial in order to determine the intervals over which it is increasing or decreasing. Decreasing function in calculus for a function, y = f (x) to be monotonically decreasing (dy/dx) ≤ 0 for all such values of interval (a, b) and equality may hold for discrete values. Find the derivative, f' (x), of the.

To find intervals of increase and decrease, you need to differentiate the function concerning x. Find where increasing/decreasing f (x) = square root of x. Increasing functions in the above graph, the function is increasing between the interval of (0, 2).

Find the region where the graph goes down from. The increasing and decreasing nature of the functions in the given interval can be found out by finding the derivatives of the given function. Q’ =.5 (k*m)* (l*m) =.5*k*l*m 2 = q * m 2.

Now perform the second derivation of f (x) i.e f”. Take a quadratic equation to compute the first derivative of function f' (x). First, find the derivative of f(x) f ( x).