For now, we will stick with relative extrema.

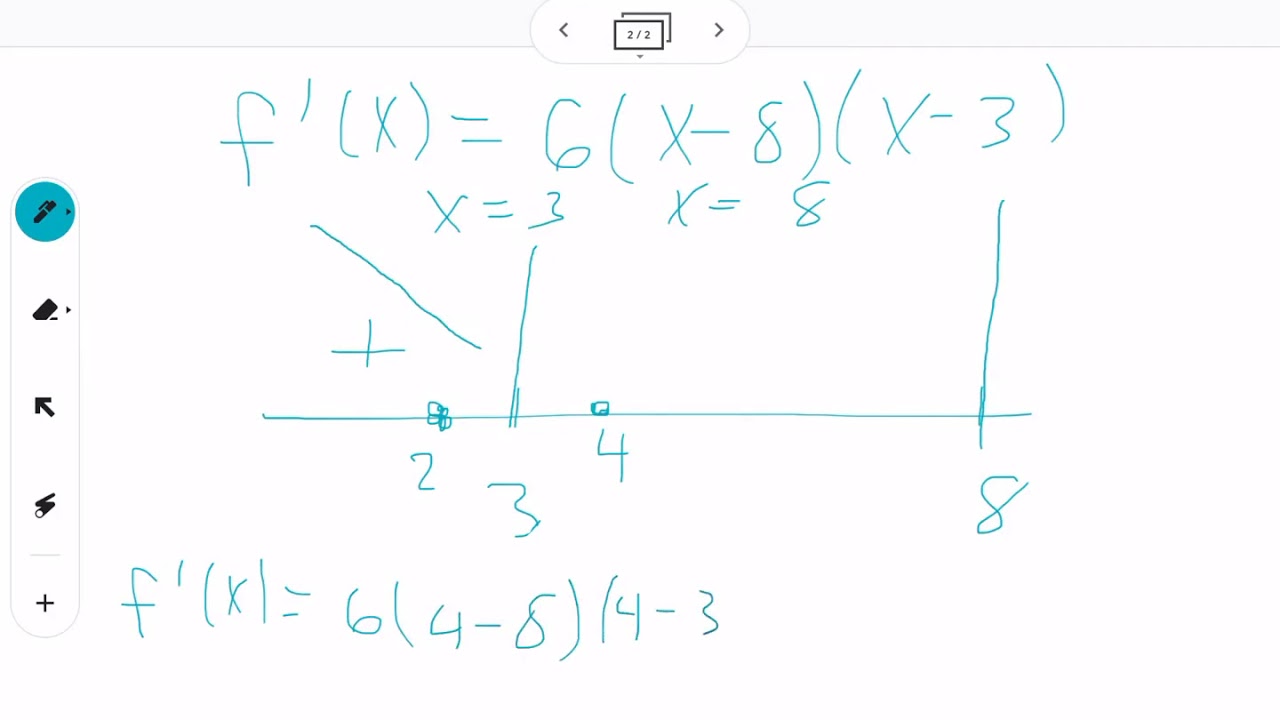
Finding relative extrema. Finding the global minimum local extrema (relative extrema) local extrema are the smallest or largest outputs of a small part of the function. Now we substitute the critical number and both endpoints into the function to determine absolute extrema. To nd and classify relative extremum we follow a procedure very similar to what we did to nd intervals where a function increases/decreases.

Find all relative extrema and saddle points of the function f(x, y) = x 2 − xy − y 2 − 3x − y. Using the first derivative test to find relative. We will find the relative extrema for ω ( t) = ( x − 1) 2 ( x − 3) 2 3 🔗 using three different methods.

Since the derivative of a function is the slope, we. Fun‑4 (eu), fun‑4.a (lo), fun‑4.a.2 (ek) google classroom facebook twitter. Because a relative extremum is extreme locally by looking at points close to it, it is.

A value c ∈ [. Math calculus applications of derivative. How do we find relative extrema?

Now, follow the given steps to find its points of relative extrema: For a given function fwe: Finding absolute extrema of f (x) f ( x) on [a,b] [ a, b] verify that the function is continuous on the interval [a,b] [ a, b].

An absolute minimum is the lowest point of a function/curve on a specified interval. F ( 3) = 3 ( 3) 2 − 18 ( 3) + 5 = − 22 f ( 0) = 3 ( 0) 2 − 18 ( 0) + 5 = 5 f. Collectively maxima and minima are known as extrema.