Asymptote in geometry, a line associated with a curve in such a way that the distance between.

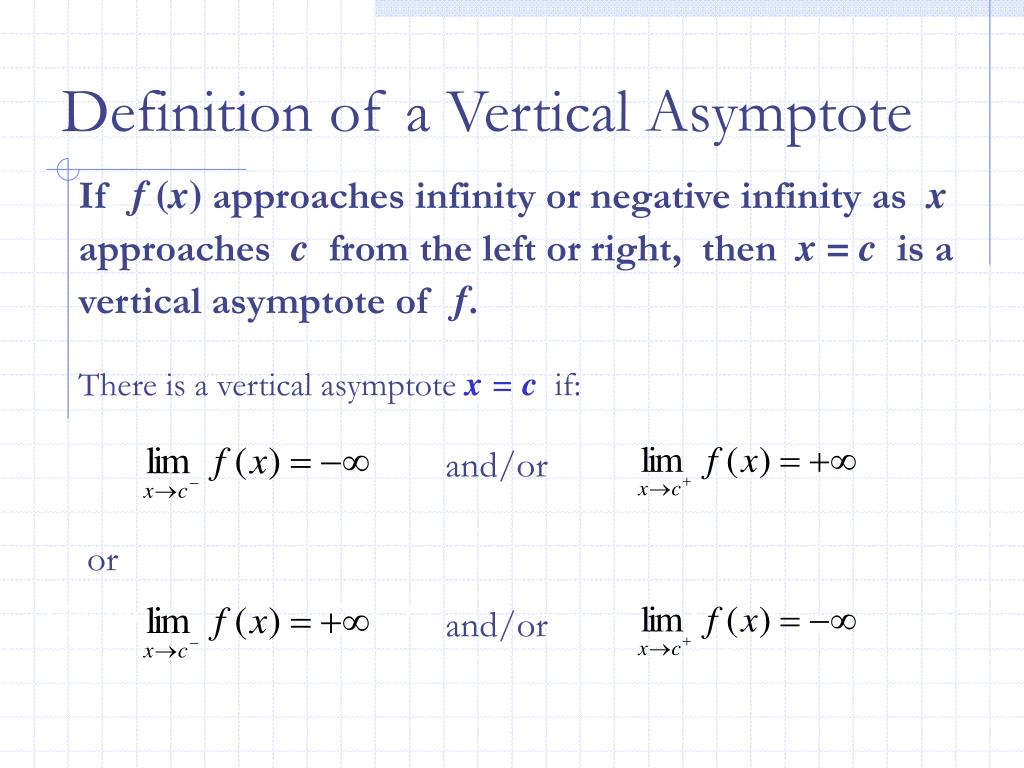
Definition vertical asymptote. Degree of numerator = 1. Vertical asymptote when x approaches some constant value c from left. Asymptote ( ˈæsɪmˌtəʊt) n (mathematics) a straight line that is closely approached by a plane curve so that the perpendicular distance between them decreases to zero as the distance from.

Vertical asymptotes it is a vertical asymptote when: (redirected from vertical asymptote) also found in: Vertical asymptote rules when the graph gets closer to the vertical asymptote, it tends to negative/ positive infinity.

Vertical asymptotes are straight lines of the equation , toward which a function f ( x) approaches infinitesimally closely, but never reaches the line, as f ( x) increases without bound. A removable discontinuity is a hole along the curve of a function in a rational function graph. Asymptotes have a variety of.

Degree of denominator = 2. A vertical asymptote is an area of a graph where the function is undefined. Since the degree of the numerator is smaller than that of the denominator, the horizontal asymptote is given by:

The graph will approach this line, but it won’t dare touch or cross it. In analytic geometry, an asymptote (/ˈæsɪmptoʊt/) of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the x or y coordinates tends. The distance between asymptote and graph.

An asymptote is defined as a straight line that approaches a curve. Vertical asymptotes are invisible or ghost lines that show where a rational function is not allowed. The vertical asymptote is a place where the function is undefined and the limit of the function does not exist.