What is the definition of quotient of powers?

Quotient of powers definition. Similarly, the quotient rule tells us how to find the derivative of a function, f(x), that is the ratio of two differentiable functions, u(x) and v(x): The power of a quotient is equal to the quotient obtained when the dividend and divisor are each raised to the indicated power separately, before the division is. In this tutorial you'll see how exponents add when you divide the same number raised to different.

The law is stated as follows: Learn how this rule works along with examples in simple and complex division. The quotient formula is given as follows:

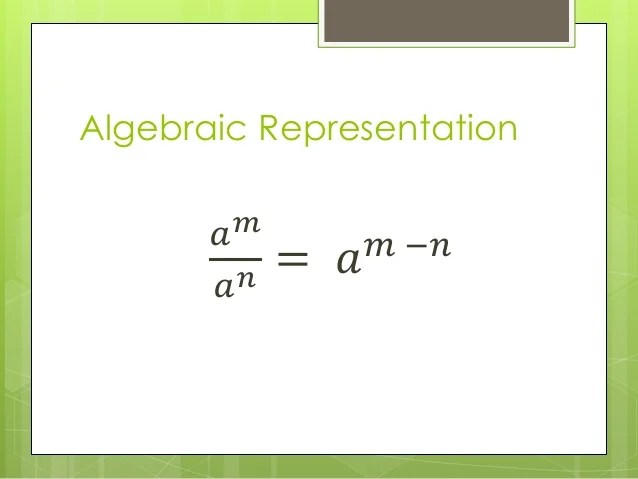
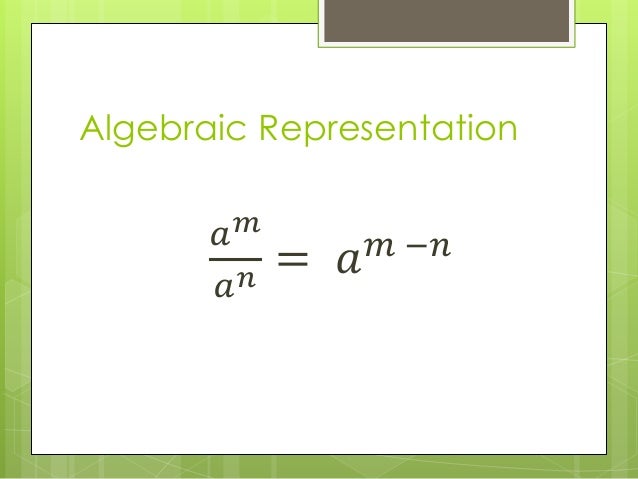
Below are two examples of the quotient of powers theorem in effect: When you have a number or variable raised to a. The quotient of powers of same base is equivalent to the same base to the power of the difference of exponents:

We can derive the quotient rule from first. The quotient of powers rule is used to simplify the problem of division that involves exponents. In order to use this formula, the bases must be the same.

The power of a quotient rule states that the power of a quotient is equal to the quotient obtained when the numerator and denominator are each raised to the indicated power. When you multiply two powers with the same base, you add the exponents. The first step will consist of checking that each of the powers that participate in the division coincide fully, in reference to their numerator and denominator.

What is the definition of a quotient of powers? Quotient of powers is a formula that lets us simplify terms with exponents that need to be divided. Dividend ÷ divisor = quotient +.