Definition we say that x = c x = c is a critical point of the function f (x) f ( x) if f (c) f ( c) exists and if either of the following are true.

Critical points of a graph. Critical points and relative extrema.critical points are the points on the graph where the function’s rate of change is altered—either a change from increasing to. Critical points are most often used to plot functions, and using the graphs below to gain an intuition about the behavior of critical points will be useful in the future when having to. Note that a couple of the problems involve equations that may not.

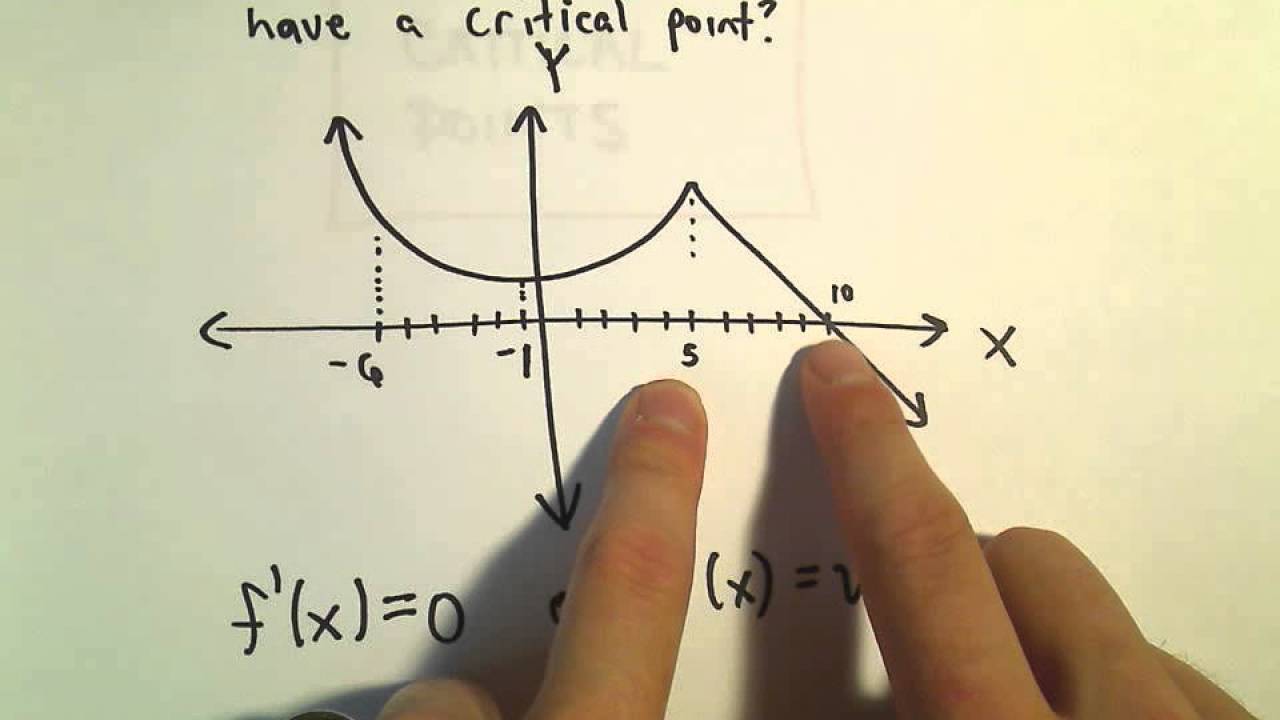
Recall that if f ' (x) = 0 or f ' (x) is undefined, there is a critical point. Critical points exist where the derivative is 0, and represent points at which the graph of the function changes direction from decreasing to increasing, vice versa. The corresponding critical value is f (0) = 0.

Critical points are used in finding the extrema and in optimization problems. Critical points are key in calculus to find maximum and minimum values of graphs. Mark out those points and trace.

Period b note that the distance between the points: While it is critical to understanding a graph, we do not have a specific point in this case. If a critical point is neither of the above, then it signifies a vertical tangent in the graph of a function.

First derivative as slope to understand critical points, we need have a. We know that critical points are the points where f'. Just a quick example of finding critical points from a given graph.

X = 1 and y = 4 so our critical point is (1,4) x =. The absolute value function f (x) = |x| is differentiable everywhere. The graph of the function f has a cusp at this point with vertical tangent.