On the graph, the critical points are the points where the rate of change of function is altered.

Critical point on a graph. As the complexity of the functions increase, we see more and more complex behavior from their graphs, and it becomes harder to graph. The triple point represents a temperature and pressure combination where all three states of matter exist. The main point of this section is to work some.

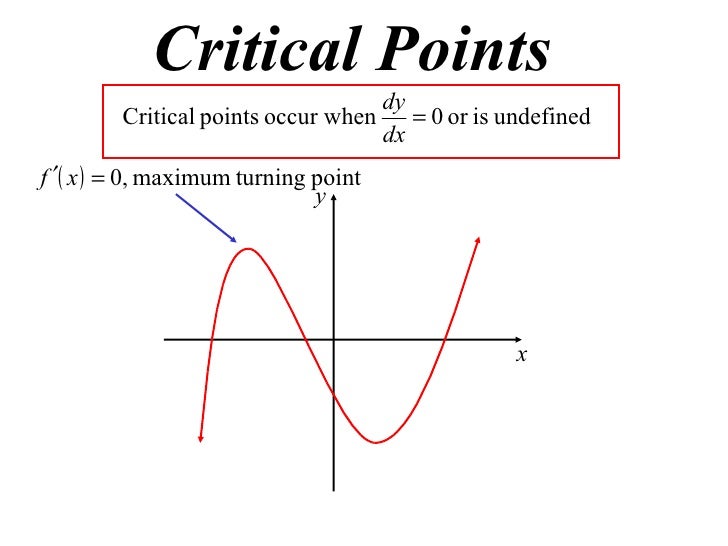
Critical point (32.17 °c, 48.72 bar), opalescence. Thanks to all of you who support me on patreon. Points on the graph of a function where the derivative is zero or the derivative does not exist are important to consider in many application problems of the derivative.

While all local maxima and local. So, the critical points on a graph increases or decrease, which can be found by differentiation and substituting the x value. A critical point of a function \(y=f(x)\) is the point \((c, f(c))\), at which the slope of the graph is zero.

In most cases, though, the graph method will not be convenient because most functions are in expressions than graphs. This particular method of finding critical points is the graph method. How to calculate a critical point?

The triple point and critical point are both found on a phase diagram. If we use a calculator to sketch the graph of a function, we can usually spot the least and. There have lots of peaks and.

If at a critical point is the derivative is equal to zero, it is called a stationary point (where. A critical point of a function of a single real variable, f ( x ), is a value x0 in the domain of f where it is not differentiable or its derivative is 0 ( f ′ ( x0) = 0). Use this online critical point calculator with steps that.